GetStudySolution
Getstudysolution is an online educational platform that allows students to access quality educational services and study materials at no cost.
NCERT Solutions for class 7 Maths chapter 10 – Practical Geometry
Back Exercise
Exercise 10.1
Question 1.
Draw a line, say, AB, take a point C outside it. Through C, draw a line parallel to AB using ruler and compasses only.
Solution:
Steps of Construction
- Draw a line AB.
- Take a point C outside it.
- Take any point D on AB.
- Join C to D.
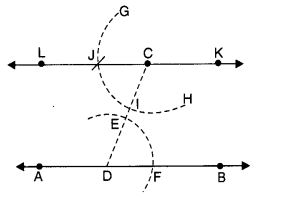
- with D as centre and a convenient radius, draw an arc cutting AB at F and CD at E.
- Now with C as centre and the same radius as in step 5, draw an arc GH cutting CD at I.
- Place the pointed tip of the compasses at F and adjust the opening so that the pencil tip is at E.
- With the same opening as in step 7 and with I as centre, draw an arc cutting the arc GH at J.
- Now join CJ to draw a line ‘KL’. Then KL is the required line.
Question 2.
Draw a line l. Draw a perpendicular to l at any point on l. On this perpendicular choose a point X, 4 cm away from l. Through X, draw a line m parallel to l.
Solution:
Steps of Construction
- Draw a line l.
- Take any point A on line l.
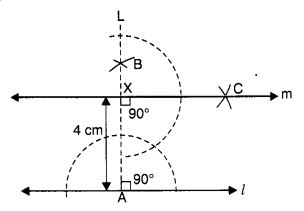
- Construct an angle of 90° at point A of line l and draw a line AL perpendicular to line l.
- Mark a point X on AL such that AX = 4 cm.
- At X construct an angle of 90° and draw a line XC perpendicular to line AL.
- Then line XC (line m) is the required line through X such that m || l.
Question 3.
Let l be a line and P be a point not on l. Through P, draw a line m parallel to l. Now join P to any point Q on l. Choose any other point R on m. Through R, draw a line parallel to PQ. Let this meet l at S. What shape do the two sets of parallel lines enclose?
Solution:
Steps of Construction
- Draw a line l and take a point P not on it.
- Take any point Q on l.
- Join Q to P.
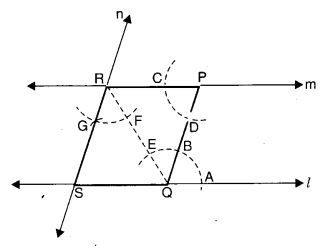
- Draw a line m parallel to line l, as shown in the figure. Then line m || line l.
- Join P to any point Q on l.
- Choose any point R on m.
- Join R to Q.
- Through R, draw a line n parallel to the line PQ.
- Let the line n meet the line l at S.
- Then, the shape enclosed by the two sets of parallel lines is a parallelogram.
Exercise 10.2
Question 1.
Construct A XYZ in which XY = 4.5 cm, YZ = 5 cm and, ZX = 6 cm.
Solution:
Steps of Construction
- Draw a line segment YZ of length 5 cm.
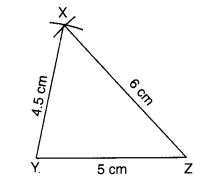
- With Y as centre, draw an arc of radius 4.5 cm.
- With Z as centre, draw an arc of radius 6 cm,
- Mark the point of intersection of arcs as X.
- Join XY and XZ. ∆ XYZ is now ready.
Question 2.
Construct an equilateral triangle of side 5.5 cm.
Solution:
Steps of Construction:
- Draw a line segment BC of length 5.5 cm.
- With B as centre, draw an arc of radius 5.5 cm.
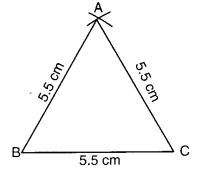
- With C as centre, draw an arc of radius 5.5 cm.
- Mark the point of intersection of arcs as A.
- Join AB and AC. Equilateral ∆ ABC is now ready.
Question 3.
Draw ∆ PQR with PQ = 4 cm, QR =3.5 cm and PR = 4 cm. What type of triangle is this?
Solution:
Steps of Construction:
- Draw a line segment QR of length 3.5 cm.
- With Q as centre, draw an arc of radius 4 cm.
- With R as centre, draw an arc of radius 4 cm.
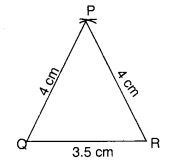
- Mark the point of intersection of arcs as P.
- Join PQ and PR.
∆ PQR is now ready,
∵ PQ = PR
∴ ∆ PQR is isosceles.
Question 4.
Construct ∆ ABC such that AB = 2.5 cm, BC = 6 cm and AC = 6.5 cm. Measure ∠B.
Solution:
Steps of Construction
- Draw a line segment BC of length 6 cm.
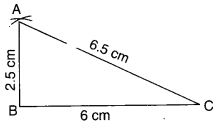
- With B as centre, draw an arc of radius 2.5 cm.
- With C as centre, draw an arc of a radius of 6.5 cm.
- Mark the point of intersection of arcs as A.
- Join AB and AC.
- ∆ ABC is now ready. On measurement, ∠B = 90°.
Exercise 10.3
Question 1.
Construct ADEF such that DE = 5 cm, DF 3 cm, and m ∠EDF = 90°.
Solution:
Steps of Construction:

- Draw a line segment DE = 5cm.
- Draw ∠EDX = 90°.
- With centre D and radius = 3 cm, draw an arc to intersect DX at F.
- Join EF to obtain the required triangle DBF.
Question 2.
Construct an isosceles triangle in which the length of each of its equal sides is 6.5 cm and the angle between them is 110°.
Solution:
Steps of Construction
- Draw a line segment QR of length 6.5 cm.
- At Q, draw QX making 110° with QR, using a protractor.
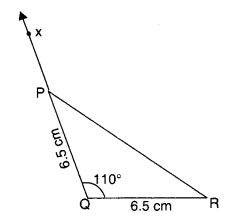
- With Q as centre, draw an arc of a radius of 6.5 cm. It cuts QX at P.
- Join PR. ∆ PQR is now obtained.
Question 3.
Construct ∆ ABC with BC = 7.5 cm, AC = 5 cm and m ∠C = 60°.
Solution:
Steps of Construction:
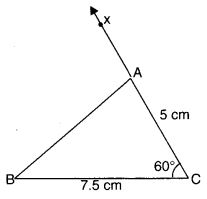
- Draw a line segment BC = 7.5 cm.
- Draw ∠BCX = 60°.
- With C as centre and radius = 5 cm, draw an arc intersecting CX at A.
- Join AB to obtain the required ∆ABC.
Exercise 10.4
Question 1.
Construct ∆ ABC, given m ∠A = 60°, m ∠B = 30° and AB = 5.8 cm.
Solution:
Steps of Construction
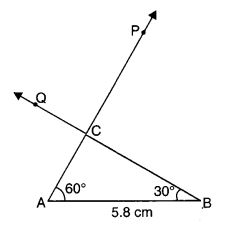
- Draw AB of length 5.8 cm.
- At A, draw a ray AP making an angle of 60° with AB.
- At B, draw a ray BQ making an angle of 30° with BA.
- Mark the point of intersection of two rays as C.
- ∆ ABC is now completed.
Question 2.
Construct ∆ PQR if PQ = 5 cm, m ∠PQR = 105° and m ∠QRP = 40°.
(Hint: Recall angle-sum property of a triangle).
Solution:
By angle-sum property of a triangle
m ∠RPQ + m ∠PQR + m ∠QRP = 180°
⇒ m ∠RPQ + 105° + 40° = 180°
⇒ m ∠RPQ + 145° = 180°
⇒ m ∠RPQ = 35°
Steps of Construction
- Draw PQ of length 5 cm.
- At Q, draw a ray QX making an angle of 105° with QP.
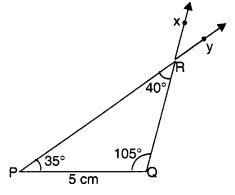
- At P draw a ray PY making an angle of 35° with PQ.
- Mark the point of intersection of two rays as R.
∆ PQR is now completed.
Question 3.
Examine whether you can construct ∆DEF such that EF = 7.2 cm, m ∠E = 110° and m ∠F = 80°. Justify your answer.
Solution:
m ∠E + m ∠F = 110° + 80° = 190° > 180°
This is not possible since the sum of the measures of the three angles of a triangle is 180°. As such, the sum of two angles of a triangle cannot exceed 180°.
Hence, ∆ DEF cannot be constructed.
Exercise 10.5
Question 1.
Construct the right-angled ∆ PQR where m ∠Q = 90°, QR = 8 cm and PR = 10 cm.
Solution:
Steps of Construction:
- Draw QR of length 8 cm.
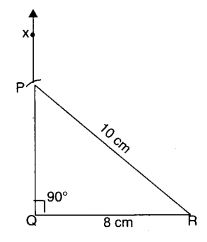
- At Q, draw QX ⊥ QR.
- With R as centre, draw an arc of radius 10 cm.
- Mark the meeting point of these two as P.
∆ PQR is now obtained.
Question 2.
Construct a right-angled triangle whose hypotenuse is 6 cm long and one of the legs is 4 cm long.
Solution:
Steps of Construction
- Draw QR of length 4 cm.
- At Q, draw QX ⊥ QR.
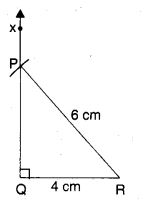
- With R as centre, draw an arc of radius 6 cm.
- Mark the meeting point of arc and QX as P.
∆ PQR is now obtained.
Question 3.
Construct an isosceles right-angled triangle ABC where m ∠ACB = 90° and AC = 6 cm.
Solution:
Steps of Construction
- Draw AC of length 6 cm.
- At C, draw CX ⊥ CA.
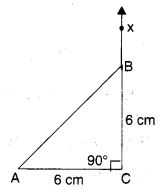
- With C as centre, draw an arc of radius 6 cm to intersect CX at B.
- Join AB. ∆ACB is now obtained.